how do you test orthopedic implants?
Metals are my favorite materials to work with because they're so well understood. They’ve been around for a very long time and they have well documented and predictable properties. If you’re designing a simple metal part and you want to verify how it will perform under load you can simply look up the material properties and run the hand calcs to find out.
Nothing to it.
If the part geometry gets more complex, analysis is still really straightforward. You just create an approximate model (or mesh) of the part, apply the very well documented properties of your chosen material to the elements and apply the loads you expect the part to encounter.
If you want to be absolutely sure your part will work (and you have the budget), you build a bunch of prototypes and load them until they break.
The mills that make the metals are so tightly controlled that you can be 99.9999% confident [citation needed /s] that the material in your actual part will perform exactly the same as the ones you broke in prototyping. And when metal parts break...they stay broken (1).
The materials in orthopedic implants though, are way different! Well...half of them are.
The titanium behaves like a well-defined metal should. It deflects and rebounds predictably in the elastic range, it has well-known tensile and yield strength...all things you want in an engineering material. But the bone the implant is embedded in? Way less predictable.
This presents the orthopedic device engineer with a unique set of limitations: Prototyping is challenging (2). Turns out, it's really hard to get people to volunteer to have implants embedded and snapped off under high load to gather test data. [citation needed /s]
Hand calcs don't yield useful results and conventional finite element models can't capture the local failure of the bone effectively.
So how do you verify that the bone the implant is embedded in will hold up under pressure?
Sloan Kulper and his team at Lifespans have been working hard to do exactly that with a mathematical modeling technique called particle-based-analysis. Unlike conventional finite element analysis where the structure is represented by a mesh of elements with known properties, particle-based analysis relies on (wait for it...) particles.
These mathematically defined particles have intrinsic material properties much like the elements in an FEA model, but unlike FEA, each particle can have different properties
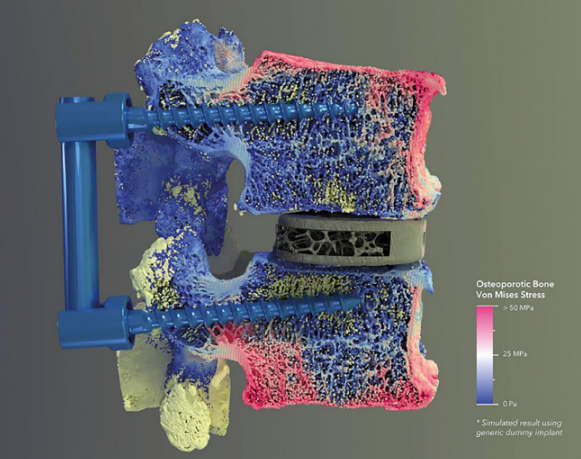
A fracture can be represented accurately without causing the model to fail and large deformations are not a problem. Sloan and his team have developed a particle based analysis tool called Alfonso that leverages particle based analysis to create and test incredibly accurate models of orthopedic implants as well as the surrounding bone tissue.
Alfonso accounts for all of the variability in the bone density, local tensile strength, deformation after implant insertion and creates a model of how the bone portion of the orthopedic device will behave under load. Alfonso’s particle based approach allows engineers to simulate damage in the bone.
In machinery design damage=failure. In orthopedic implants, damage is just part of the process. The stresses and strains involved in seating the implant signal the body to heal the damaged areas. Damage is not failure, it’s an expected outcome that needs to be understood in order to be effectively mitigated.
In conventional finite element analysis, the elements between two nodes are the driver of the analysis result. They deform according to well-established material properties in the elastic range. But what happens when the elastic stress of the material is exceeded? What if the part breaks? Here is where conventional FEA falls flat.
In order to model a fracture, an element would need to be split into two pieces and new elements would need to be added to close the mesh. Alfonso’s particle based simulation avoids this issue entirely and allows simulation of the cracking and crushing that is normal in an orthopedic implant site.
This accurate simulation of the bone portion of the orthopedic implant joint allows engineers to dramatically reduce product development timelines, and no one has to volunteer to be a test specimen!
—————————————————————————————————————————
- Exception that proves the rule: https://news.mit.edu/2013/tension-can-fuse-metal-1009
- Apparently there’s a whole industry dedicated to making artificial bones for testing, but they’re all uniform density blocks so the point stands! https://www.sawbones.com/